The importance of a statement of conformity

Minerva Metrology and Calibration lives up to our promise to ensure our clients a carefree traceability in the calibration process. This article elaborates about the importance of a statement of conformity, and the ILAC-G8:09/2019 Guidelines on Decision Rules and Statements of Conformity that Minerva implemented recently.
A statement of conformity is an integer part of the daily activities at Minerva. Every day we make a lot of decisions based on the observations, measurements and the rules (acceptance criteria) in metrology. Since the implementation of the new version of the ISO/IEC 17025:2017 standard, two issues occurred that have become the most discussible improvements in this version of the standard. These are the statement of conformity and decision rules.
Technical aspects of conformity statement
Conformity statement, as broadly defined, is any activity undertaken to determine, directly or indirectly, whether a product, process, system, person or body meets relevant standards and fulfills specified requirements.
In a particular kind of conformity statement, the determination that a product fulfils a specified requirement relies on measurement as a principal source of information. This approach includes explicit consideration of risks and develops general procedures for deciding about conformity based on measurement results. Also the approach recognizes the central role of probability distributions as expressions of uncertainty and incomplete information.
In conformity assessment, a measurement result is used to decide if an item of interest conforms to a specified requirement. The item might be, for example, a pressure gauge or pressure transmitter to be calibrated in compliance with ISO/IEC 17025:2017. The requirement typically takes the form of one or two tolerance limits that define an interval of permissible values of a measurable property of the item. This is also called a a tolerance interval. Examples of such properties include the error of indication of a pressure gauge, pressure transmitter, long-term drift, et cetera. If the true value of the property lies within the tolerance interval, it is said to be conforming, and non-conforming if it lies outside the tolerance interval.
In general, deciding whether an item conforms will depend on a number of measured properties and there might be one or more tolerance intervals associated with each property. There may also be several possible decisions with respect to each property, given the result of a measurement. In the evaluation of measurement data, knowledge of the possible values of a measurand is, in general, encoded and conveyed by a probability density function, or a numerical approximation of such a function.
In a typical measurement, the measurand of interest itself is not observable. The error of indication of a pressure gauge, for example, cannot be directly observed, but one could observe the indication of its scale while reference pressure is applied. Such an indication conveys information about the pressure through a measurement model that includes the effects of influence quantities such as a reference standard, environmental conditions et cetera. In conformity assessment, an accept/reject decision is based on observable data (measured quantity values, for example) that lead to an inference regarding the possible values of a non-observable measurand.
Because of uncertainty in measurement, there is always the risk of incorrectly deciding whether an item conforms to a specified requirement based on the measured value of a property of the item. Such incorrect decisions are of two types: an item accepted as conforming may be non-conforming, and an item rejected as non-conforming may be conforming.
Why is conformity statement so important?
There is a good example which explains the importance of conformity statement more than any other argument.
In highway law enforcement, the speed of motorists is measured by police using devices such as radars and laser guns. A decision to issue a speeding ticket, which may potentially lead to an appearance in court, must be made with a high degree of confidence that the speed limit has actually been exceeded.
Using a particular Doppler radar, speed measurements in the field can be performed with a relative standard uncertainty u(v)=v of 2 % in the interval 50 km/h to 150 km/h. Knowledge of a measured speed v in this interval is assumed to be characterized by a normal distribution (PDF) with expectation v and standard deviation 0,02v. Under these conditions one can ask, for a speed limit of v0 = 100 km/h, what threshold speed vmax (acceptance limit) should be set so that for a measured speed v > vmax the probability that v > v0 is a least 99.9 %?
This mathematical problem is equivalent to the calculation of a conformance probability for a one-sided tolerance interval. Here a value of z = (vmax > v0)/(0,02vmax) is needed for which 99.9 % of the probability lies in the region V > v0. For this level of confidence z = 3,09, so that
The interval [100 km/h < v < 107 km/h] is a guard band that ensures a probability of at least 99.9 % that the speed limit has been exceeded for a measured speed of 107 km/h or higher. If you drive slower than 107 km/h you’ll not be fined for speed limit exceeding.
Minerva assists laboratories
Last year Minerva implemented the ILAC-G8:09/2019 Guidelines on Decision Rules and Statements of Conformity. We prepared a guidance document to assist laboratories in the use of decision rules when declaring statements of conformity to a specification or standard as required by ISO/IEC 17025:2017. Our laboratory decided to select a Non-binary Statement with Guard Band as a one of the possible rules.
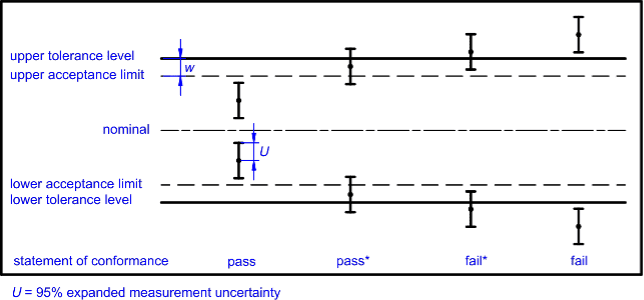
Decisions are based on guard banded acceptance limits ( = , AL = TL - w) where U is the expanded measurement uncertainty calculated according to the Guide to the Expression of Uncertainty of Measurement (GUM). AL and TL – acceptance and tolerance limits respectively.
The estimate of the measurand is assumed to have a normal probability distribution and a specific risk is used for the risk calculation. In this case the risk of accepted items to be outside the tolerance limit is < 2.5%. For rejected items the risk to be inside the tolerance limit is < 2.5%. When the measured result is close to the tolerance, the risk of false accept and false reject raises up to 50%. Measurement results with limitations according to ILAC-G8:03/2019 are marked as stated in the visual below:
pass - The measured values were observed in tolerance at the points tested. The specific false accept risk is up to 2.5%.
pass* - The measured values were observed in tolerance at the points tested. However, a portion of the expanded measurement uncertainty intervals about one or more measured values exceeded tolerance. When the measured result is close to the tolerance, the specific false accept risk is up to 50%.
fail* - One or more measured values were observed out of tolerance at the points tested. However, a portion of the expanded measurement uncertainty intervals about one or more measured values were in tolerance. When the measured result is close to the tolerance, the specific false reject risk is up to 50%.
fail - One or more measured values were observed out of tolerance at the points tested. The specific false reject risk is up to 2.5%.
n/a - it's not possible to determine conformance with specification (U > TL).
Conclusions
It is the responsibility of a laboratory to agree with its customer on a service that includes decision rule documentation and application. It is important that the request for a statement of conformity comes from the customer. However, the Minerva laboratory may offer standard service deliverables with differing amounts of guard band (including zero) to provide our customer different choices of levels of risk. If there is no specification from the customer, we apply Non-binary Statement with Guard Band and publicly available factory specification (technical data sheet, standards et cetera). The appropriate acceptance criteria and decision rules may impact significantly on the production process and allow to save money.
Minerva trusts that by implementing the ILAC-G8:09/2019 Guidelines on Decision Rules and Statements of Conformity we live up to ensure you a carefree traceability in the calibration process.
November 27, 2024